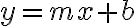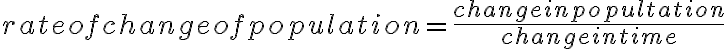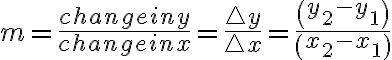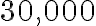UniSA Online - Online Experience

Topic outline

• Linear Equations: Sketching

  Prev Next
  • This section will provide you with an opportunity to draw a sketch of the equation of the line in order to understand the effect of change in the value of the slope of a line and its vertical intercept. 

    • ---

      Watch

      Linear Functions and Forms of Lines: Part B (21m 24s)
    • MATH 1076 - Linear functions and forms of lines_ Part B_default_0856aa5b

      
      

      
      
      
      
    • ---

       Linear Functions

      Linear functions describe quantities that have a constant rate of increase (or decrease). A linear function is a function that can be written:

      $f\left( x\right) =mx+b$

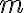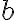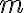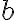      for real-valued constants $m$ and $b$. We call the constants $m$ and $b$ parameters for the family of linear functions.

      We can also call the output of the function 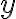$y$, in which case we describe our family of linear functions as:

      $y =mx+b$
      

      Recall: We solved linear equations in a previous week. Now we are investigating the properties of the whole family of linear functions. Being able to solve linear equations is a useful skill in exploring linear functions.

      
      
    • Activity 4M - Linear Functions

      What
      This activity lets you practice applying your knowledge of linear functions.

      How

      • Enter the correct responses to each question in the space provided.

      
      
      
      

      Now, complete the graph below to show the population as indicated by the linear equation $P(t)=30000+2000t$. Note, you do not have to share this graph, however, you should save a copy as we will be using it in the subsequent activities. 

       

      
      

    • Based on the graph in Activity 4M, we observe several features in linear functions:
      

      1. The graph is a straight line
      2. The rate of change is constant

      These are properties of all linear functions and give rise to several important features of this family of functions. 

    • ---

       Gradient

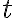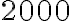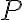      We observed in Activity 4M that a unit increase in $t$ corresponds to an increase of $2000$ in $P$. That is, each year the population increases by 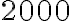$2000$. Because the rate of change of a linear function is constant, we can use any two points to find the rate of change.

      For this example, the rate of change of population is given as follows:

      $rate~of~change~of~population = \frac {change~in~popultation}{change~in~time}$

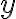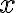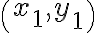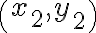      In general, the rate of change, also called gradient or slope, is the change in $y$ per unit change in $x$. Given any two points $\left( x_{1},y_{1}\right)$ and $\left( x_{2},y_{2}\right)$, the gradient 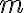$m$ is found by using:

      $m=\dfrac {change~in~y}{change~in~x}=\dfrac {\triangle y}{\triangle x}= \dfrac {\left( y_{2} -y_{1}\right)}{\left( x_{2}-x_{1}\right) }$

      This can be seen diagramatically below:

      
      

      We say that changes in the value of 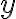$y$ are proportional to changes in the value of 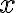$x$. The valye 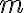$m$ is sometimes called the constant of proportionality. 

    • Activity 4N - Gradient

      What
      Use this activity to self-check you knowledge of gradient. 

      How

      • To complete this activity, you will need your completed graph from Activity 4M.
      • Answer the question below.
    • Activity 4O - Gradient as a Rate of Change

      What
      Use these activities to self-check your knowledge of gradient as a rate of change. 

      How

      • This activity has two parts. In part A you need to match the functions with their correct graph (you may wish to use a table of values to assist). In part B, drag and drop the text to complete the statements about slope. 

      Part A:

      
      

      Part B:

    • ---

        Vertical Intercept

      We observed in Activity 4M that when the independent variable $t$ is zero, the initial value is $30,000$. In general, the constant $b$ tells us the vertical intercept (often called the $y$-intercept) of the function.

    • Activity 4P - Vertical Intercept

      What
      This activity gives you the opportunity to check your knowledge of the vertical intercept as it relates to linear functions. 

      How

      • This activity has two parts. In part A you need to match the functions with their correct graph (you may wish to use a table of values to assist). In part B, select the correct answer to the questions about slope. 

      Part A:

      
      

    Mark as done
  • 

    Activity 4M - Linear Functions Interactive Content

    Mark as done
  • 

    Activity 4N - Gradient Interactive Content

    Mark as done
  • 

    Activity 4O - Gradient as a Rate of Change: part a Interactive Content

    Mark as done
  • 

    Activity 4O - Gradient as a Rate of Change: part b Interactive Content

    Mark as done
  • 

    Activity 4P - Vertical Intercept: part a Interactive Content

    Mark as done
  • 

    Activity 4P - Vertical Intercept: part b Interactive Content

    Mark as done

  Prev Next